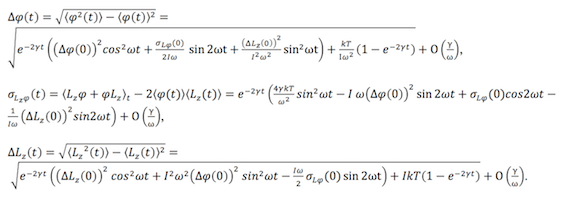
Cover Image

THE FIRST AND SECOND MOMENTS FOR THE QUANTUM BROWNIAN PLANAR ROTATOR IN EXTERNAL HARMONIC CLASSICAL FIELD

Igor S. Petrović, Jasmina M. Jeknić-Dugić

DOI Number
10.2298/FUPCT1702071P
First page
071
Last page
079

Abstract


We derive explicit expressions for the first and second moments as well as the correlation function for a planar (one-dimensional) quantum Brownian rotator placed in the external harmonic potential. Our results directly provide the standard deviations for the azimuthal angle and the canonically conjugate angular momentum for the rotator. We find that there are some significant physical differences between this model and the free rotator model, which is well investigated in the literature.


HIGHLIGHTS

  • Expressions were derived for the first and second moments, and the correlation function for a planar (one-dimensional) quantum Brownian rotator placed in the external harmonic potential.
  • In comparison with the well-known free Brownian rotator, we observe the existence of the stationary state in the asymptotic limit ().
  • Significantly smaller standard deviations and the correlation-function for the rotator exerted to the external harmonic potential we established.

Keywords

open quantum systems, quantum Brownian motion, quantum rotator

Full Text:

PDF

References


Boyke Schönborn, J., Rainer Herges, J., Hartke, B., 2009. J. Chem. Phys. 130, 234906

Breitenberger, E., 1985. Found. Phys. 15, 353

Breuer, H.-P., Petruccione, F., 2002. The Theory of Open Quantum Systems, Clarendon Press, Oxford.

Browne, W., Feringa, B.L., 2006. Nature Nanotech. 1, 25-35

Caldeira A.O., Leggett A.J., 1983 Physica.A 121, 587-616

Deck,. R. T., Ozturk, N., 1994. Found. Phys. Lett. 7, 419

Dugić, M., 2004, Decoherence in the classical limit of quantum mechanics, SFIN XVII(2), Institute of Physics, Belgrade (in Serbian)

Giulini D., Joos E., Kiefer C., Kupsch J., Stamatescu I.-O., Zeh H. D., 1996, Decoherence and the Appearance of a Classical World in Quantum Theory, Springer, Berlin

Hutchison J.A., et al, 2014, Nature Nanotech. 9, 131–136

Jones, R.A., 2008, Soft Machines: Nanotechnology and Life, Oxford University Press

Jordan, P., 1927. Z. Phys. 44, 1

Korobenko A.., Milner A. A., Milner, V., 2014. Phys. Rev. Lett. 112, 113004

Kottas, G.S.,Clarke, L.I.,Horinek, D., Michl, J.2005. Chem. Rev. 105, 1281-1376

Milburn, G.J., 1997, Schrodinger's Machines: The Quantum Technology Reshaping Everyday Life, W. H. Freeman

Nagle, R.K., Saff, E.B., Snider,A.D., 2011. Fundamentals of differential equations, Pearson (8th edition)

Rivas, A., Huelga, S.F., 2011. Open Quantum Systems. An Introduction, SpringerBriefs in Physics, Berlin.

Suzuki Y., Tanimura Y, J. Phys. Soc. Japan 70, 1167 (2001)


Refbacks

  • There are currently no refbacks.


ISSN 0354-4656 (print)

ISSN 2406-0879 (online)